
A critical part to ensuring your students learn math is to get them talking about math. This can be a tough task, but when math class is organized with purpose, even the students most reticent about math can find a way to engage. Whether it’s your first or your fifteenth year teaching, you will want to ensure that student interactions are structured, your class time is well managed, and classroom relationships promote productive teaching and learning. The effect can be far reaching. Establishing the right routines can serve to get students into content quicker and catch them up if they're behind—an especially important benefit given this past school year.
One way to support routines in the classroom is through math talk moves. Talk moves can be used flexibly throughout class time as a way to introduce new problems and facilitate student discourse. We encourage you to keep in mind the following ideas for promoting effective conversations. They not only work in physical classrooms but can work over webcam or in some cases even remotely with limited internet access.
Revoicing
Revoicing can help students clarify and share their thinking. It involves the teacher repeating all or part of what a student says and then asking whether the idea was stated correctly.
- “So you’re saying that the two expressions are equivalent.”
- “You’re showing how the product of the two fractions is less than 1, correct?”
As the teacher, this gives you time to think about what the student is saying and check your own understanding. Critically, it also gives other students a chance to hear the idea again, however stated more clearly and with more precise language. It is not always useful to correct mathematical language as students are in the process of learning it. However, this talk move lets you model the correct language while still honoring the idea behind what a student said.
A version of this talk move is possible remotely with limited internet access. Give students a math problem and task them with writing or making an audio or video recording of what they’re thinking. Reply and revoice using the same medium. Ideally, do this in a way that other students can see or hear you modeling precise mathematical language, such as replying in an online forum that all students have access to.
Repeating
Also considered a talk move, repeating is similar to revoicing. In this case, students restate someone else’s reasoning. When called upon, students might say “I didn’t hear” or “I didn’t understand,” but they must ask the first student to repeat his or her point and follow up by repeating what they heard.
- “Who can put that into your own words?”
- “What's another way to say what _____ said?”
This talk move slows down the discussion and gives students time to work with the ideas that other students are producing. By asking several students to repeat or restate someone else’s idea, you help all students internalize it and ultimately orient themselves to the thinking of others.
If students are able to share ideas verbally, then that should happen. However, that may not be possible for all classrooms or all students this fall, and repeating can take place in an online forum if necessary. Have some or all students post a strategy or solution to a problem you give. Other students choose someone else’s post and reply by putting the same idea into their own words.
Reasoning – Why Do You Think That?
This talk move is an especially powerful way for students to explain their thinking and get better at providing evidence for their claims. As students become more used to being asked for evidence of their thinking, they will eventually dig deeper themselves without prompting.
After a student responds to a question, follow immediately with Why Do You Think That? to press for reasoning.
- “Why do you think that? What is your evidence?”
- “Why do you think that? Explain your reasons.”
This talk move can be executed remotely, even with limited internet access. After students complete an assignment or solve a problem, call attention to all or part of their thinking and ask them “Why do you think that?” Students can write down their evidence for their answer or, if possible, record it as audio or video. This is most effective when published in a way that the entire class can see or hear.
Adding On
This talk move is used to prompt students to further participate in the discussion and engage critically with the reasoning of others. This move is especially useful when a student contributes one step toward a more complex explanation.
- “Who can take that idea to another step?”
- “What would you add to _____’s response?”
This talk move invites everyone into the conversation and encourages students to continue interacting with one another’s ideas. If you are teaching remotely, this talk move can take place in an online forum, too. Pose one or more questions that are open-ended or have multiple solutions, such as “When can two expressions be sometimes the same and sometimes different?” Or “How can geometry help you understand an algebra problem?” Task students not only to post an original answer to the question, but to choose one or more answers that others posted and take their idea to the next step.
Critique, Correct, and Clarify
Critique, correct, and clarify is a language routine that can stimulate math conversations, connect learning across disciplines, and show that learning math is dependent on engaging with language.
Present a partial or broken argument, explanation, or solution method. You as the teacher can play the role of the student who produced the response and now needs help fixing it. Prompt students to identify the error(s) or ambiguity and analyze the response in light of their own understanding of the problem. Have students work both individually and in pairs to propose an improved response.

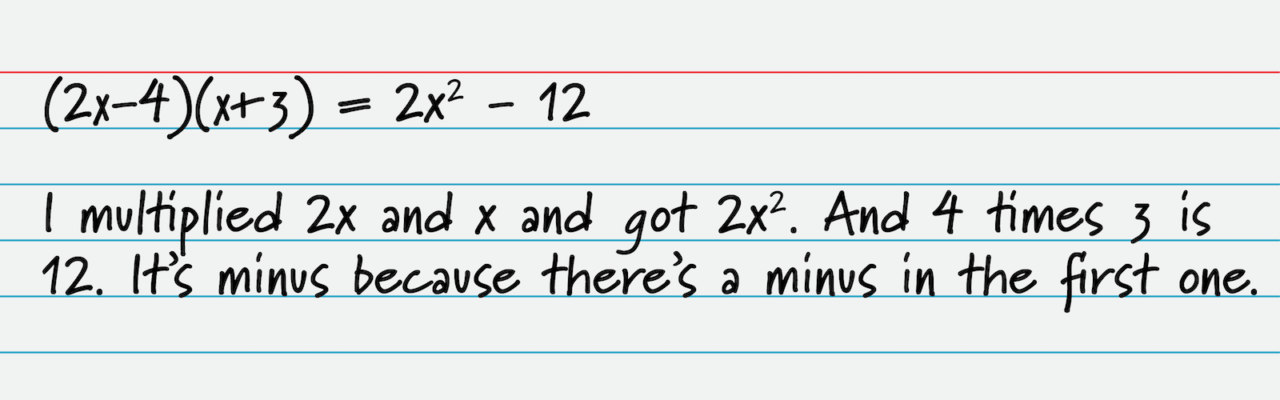
This routine gives students mathematical writing that is not their own to analyze, reflect on, and develop. The intent is for students to improve upon a flawed written argument by clarifying meaning and in effect advancing their own mathematical thinking and communication. The routine encourages students to make sense of academic content and negotiate meaning in a language to help them understand a language completely.
This routine can still be done even if you are teaching remotely to students with limited internet access. Prepare a problem that contains error(s) or ambiguity and have students write down or make an audio or video recording explaining what’s wrong with it.
* * *
Our core math solutions HMH Into Math (K–8) and HMH Into AGA (9–12) include Professional Learning Cards that provide more detail on Math Solutions' talk moves and language routines.
For more strategies to make math talk more intentional in the classroom, watch the webinar "Make Math Talk More Productive with Questioning Strategies" with Cynthia Goodman.
This blog post, originally published in 2020, has been updated for 2021.

















