
Look around you. How would you describe the shapes that make up where you live and go to school? This is one of the ways that mathematical thinking is not quite the same as numerical thinking. (Although numbers are sometimes the perfect way to describe different qualities about the shape!)
In this article, we define polygons and describe some basic ways to classify triangles and quadrilaterals. Then we provide two lessons for students in Grades 2 and up: one where students are introduced to the names for different polygons (Identifying Polygons), and one where they practice classifying triangles and quadrilaterals (Classifying Polygons).
What Is a Polygon?
When people hear the word geometry, they tend to think about shapes. Many of these shapes, or polygons, can be described as flat, closed figures with three or more sides. Polygons are two-dimensional objects, not three-dimensional solids. Encourage students to explore different ways to classify polygons. Point them towards ideas like counting sides, measuring angles, and comparing side lengths (for instance, looking for congruent sides). Many polygons have special names, which may be familiar to your students.
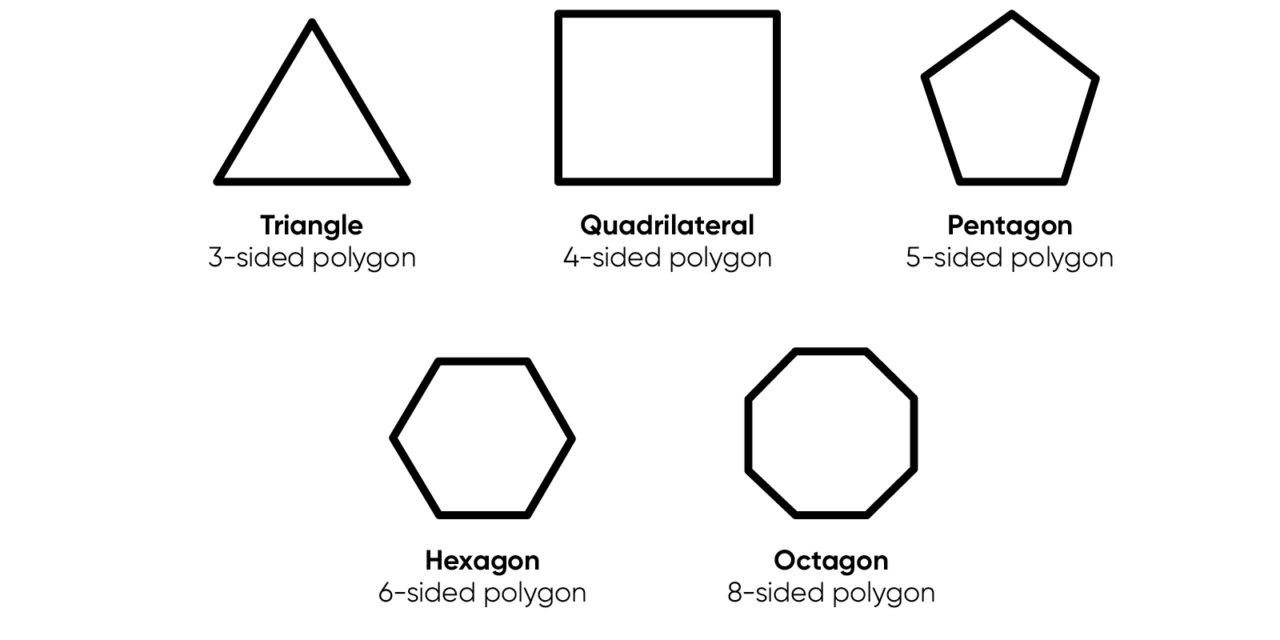
This figure shows some of the most common polygons. Notice that we identified a four-sided polygon as a quadrilateral. More commonly, you would call this polygon shape a rectangle, but a rectangle is only one kind of quadrilateral. A rectangle is a special quadrilateral where opposite sides are congruent—that is, the same length—and each angle is a right angle. A square is also a special quadrilateral because all four sides are congruent and all four angles are right angles.

What Is the Difference Between Squares and Rectangles?
Rectangles and squares are similar in many ways:
- Both are quadrilaterals (four-sided polygons).
- Both have opposite sides that are congruent.
- Both have four angles that are all right angles.
However, all four sides are congruent for a square. You could put it this way: All squares are rectangles, but not all rectangles are squares. For students who are ready, you can introduce them to our game for finding the area of rectangles.
How to Classify Triangles
Triangles have their own special cases as well. An equilateral triangle can be thought of as the square's cousin since all three sides are congruent. Another special triangle is the isosceles triangle, where only two sides are congruent. A scalene triangle has no congruent sides.

Lesson 1: Identifying Polygons
Since much of the vocabulary for polygons will be new to your students, it is a good idea to begin by making connections between objects in your classroom and new vocabulary. This will allow you to tie what the students are learning to real-life examples of polygons, along with ELA lessons.
Key Standard: Recognize shapes having specified attributes, such as a given number of angles. Identify triangles, quadrilaterals, pentagons, hexagons, and octagons. (Common Core 2.G.A.1, Florida B.E.S.T. MA.2.GR.1.1)
Materials:
- Colored paper (ideally poster paper)
- Straight edge
- Scissors
Prerequisite Skills and Concepts: Students should be able to recognize triangles and quadrilaterals by the number of sides.
Preparation: Create large versions of the following polygons by carefully using a straight edge and scissors, then post them publicly. Make sure that they are large enough for the entire class to see. If teaching remotely, use digital images or slides that all students can see and you can freely move around. Use colorful paper, and write the name of each polygon in the center.
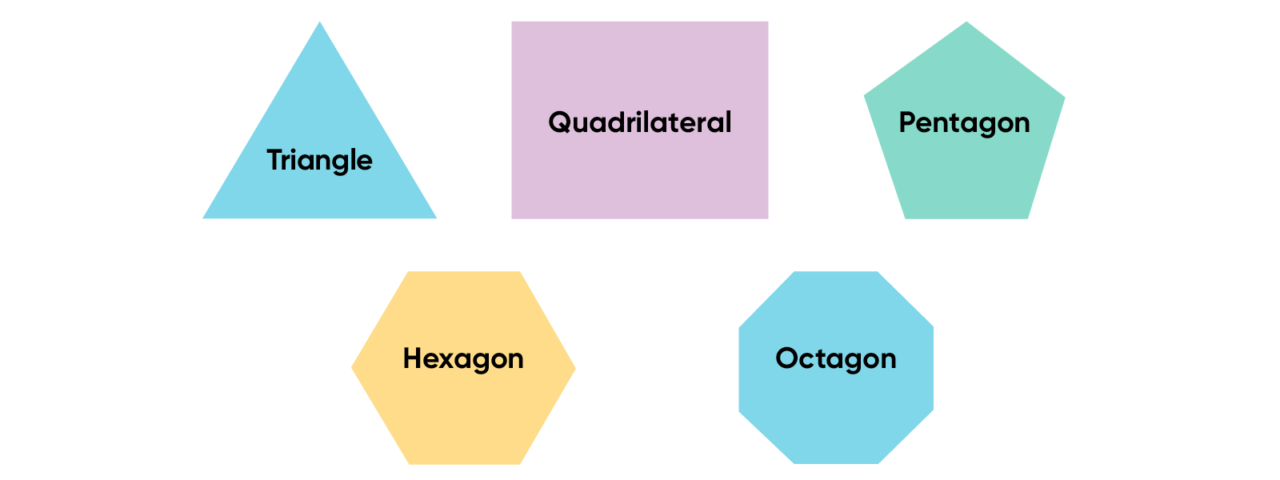
- (Point to the triangle.) Ask: What shape is this? (triangle) Can anyone tell me what makes a triangle different from other shapes?
Encourage all ideas without saying any answers are wrong. Direct students to identify a triangle as a shape with three sides. Some may also say that it has three angles. Write these properties below the polygon shape. - (Write the word tricycle publicly.) Ask: How many of you know what a tricycle is? How many wheels does a tricycle have? What do a tricycle and a triangle have in common?
When students identify that a tricycle has three wheels and a triangle has three sides, make the connection between the prefix tri- and the number three. See if any students have heard of other English words that begin with tri- and have three of something, for example triathlon, trio, trilogy, tripod, or trilingual. - Below the properties of the triangle, write "Tri means 3."
- (Point to the quadrilateral.) Ask: This shape is called a quadrilateral. What can you tell me about it?
Encourage all ideas without saying any answers are wrong. Direct students to identify a quadrilateral as a shape with four sides. Some may also say that it has four angles. Write these properties below the polygon shape. - Continue by explaining that quad- means four. Students are unlikely to know many words that begin with quad- and have four of something, but you can show examples, such as quadruple, quadrant, or quadriceps (which refers to a human muscle made up of four parts). Write "quad means 4" below the quadrilateral.
- (Pointing to the pentagon.) Say: This is a pentagon. What can you tell me about it?
Students should identify the number of sides and possibly angles of a pentagon. - Ask: Who knows what prefix means five in the word pentagon?
Explain that in this case, penta- means five. Students may be familiar with a pentathlon or the Pentagon building. - Continue by introducing the hexagon and octagon. Some students will be thinking ahead and see that the prefixes for six and eight are hexa- and octa-. Although in this lesson the prefixes are given with final vowels (e.g., octa-, not oct-), note that sometimes the prefix occurs with a different vowel (e.g., octopus) or no vowel at all (e.g., octet).
Lesson 2: Classifying Polygons
Once your students can identify different polygons, move on to identifying properties of specific polygons. This is also the time to make sure that your students know and use the correct mathematical vocabulary when describing properties of polygons.
Key Standard: Understand that shapes in different categories may share attributes, and that the shared attributes can define a larger category (e.g., quadrilaterals). (Common Core 3.G.A.1, Florida B.E.S.T. MA.2.GR.1.3)
Materials:
- Worksheet 1: Triangles and Worksheet 2: Quadrilaterals (one per student and one for you)
- Overhead projector or means of publicly displaying the worksheets
- Ruler for each student or pair of students
- Ruler to use with worksheets in front of class

Preparation: Prepare an overhead transparency of worksheets 1 and 2. Alternatively, display the worksheets on a monitor or interactive whiteboard that all students can see. Distribute the student worksheets to each child, either as printouts or digital files.
- Say: We have talked about different kinds of polygons. How did we describe a triangle?
List the properties of a triangle where all students can see: three-sided polygon, contains three angles or corners. - Say: Look at worksheet 1. All these figures are triangles, but some of them have special names. Look at figure c. Use your ruler to measure the three sides of this triangle.
Demonstrate using your own ruler. - Ask: Are all three sides the same length? (yes)
Note that people cannot measure perfectly, so students may find that some sides have slightly different lengths. Explain that the image was designed so that all sides are the same length. - Say: A triangle where all sides are the same length is called an equilateral triangle. Look at the worksheet. Are any of the other triangles equilateral? Use your ruler to check.
Allow plenty of time for students to measure, then ask for volunteers. Have students compare their reasoning without calling anyone right or wrong. Figure e is also equilateral. - Ask: Did anyone think that Figure a was equilateral?
See if any students can explain why it's not. If necessary, show that Figure a has two sides that are the same length, but the third side is a different length. - Say: A triangle with two equal sides is called an isosceles triangle. Are there any other isosceles triangles on the worksheet? Use your ruler to check.
- Say: Figure f is isosceles.
Make sure students are clear on the difference between isosceles and equilateral triangles. Usually an equilateral triangle is considered a special case of an equilateral triangle. - Say: Look at the other triangles on the worksheet. These triangles have sides that are all different lengths. These are called scalene triangles.
- You can do a similar lesson with quadrilaterals, using Worksheet 2. Have students identify rectangles and squares. Let students compare their reasoning without calling anyone right or wrong. Direct students towards identifying that squares and rectangles both have four right angles, but only squares have four congruent sides. A square is considered a special case of a rectangle.
Wrap-Up and Assessment Hints
Students need practice identifying different polygons. Have students sort groups of polygons that are oriented differently to make sure they can identify polygons however they are turned. You can also ask students to draw different polygons using a straight edge. It is also a good idea to have children draw more than one polygon of each shape using different positions. This will allow you to get a better assessment of their true understanding of the properties of each polygon.
***
Looking for a curriculum to grow student confidence in geometry, shapes, and polygons? Explore HMH Into Math, our core math solution for Grades K–8 that emphasizes the "why" behind the "how."














