For those teaching math to students who are ready to learn about absolute value, typically around Grade 6, this article presents an overview of the topic, along with two lessons to introduce and develop the concept with your students. The first lesson introduces absolute value, and the second lesson develops the concept.
What does absolute value mean?
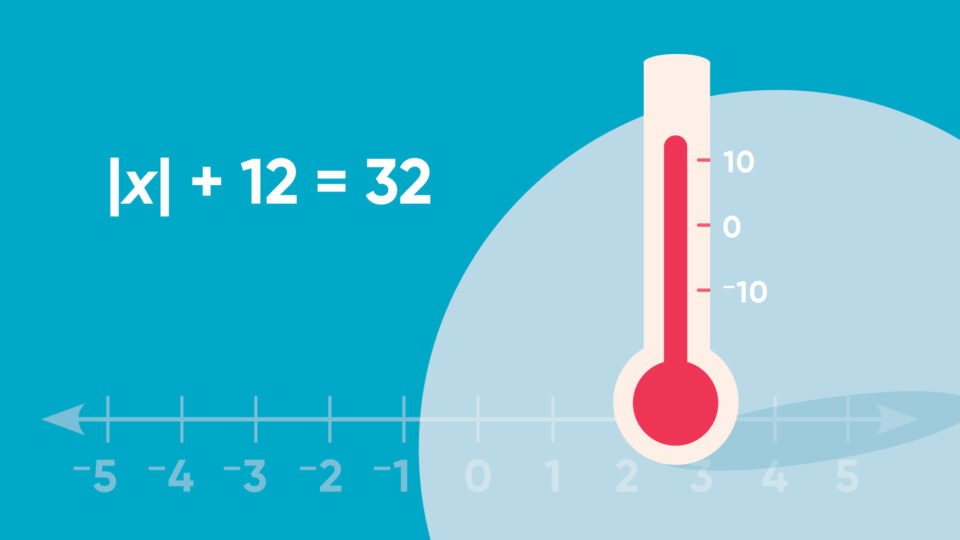
Absolute value describes the distance from zero that a number is on the number line, without considering direction. The absolute value of a number is never negative. Take a look at some examples.
- The absolute value of 5 is 5. The distance from 5 to 0 is 5 units.

- The absolute value of –5 is 5. The distance from –5 to 0 is 5 units.

- The absolute value of 2 + (–7) is 5. When representing the sum on a number line, the resulting point is 5 units from zero.

- The absolute value of 0 is 0. (This is why we don’t say that the absolute value of a number is positive. Zero is neither negative nor positive.)
Absolute value examples and equations
The most common way to represent the absolute value of a number or expression is to surround it with the absolute value symbol: two vertical straight lines.
- |6| = 6 means “the absolute value of 6 is 6.”
- |–6| = 6 means “the absolute value of –6 is 6.”
- |–2 – x| means “the absolute value of the expression –2 minus x.”
- –|x| means “the negative of the absolute value of x.”
A number line is not just a good model for showing distance from zero; it’s also a useful way to graph equalities and inequalities that contain expressions with absolute value.
Consider the equation |x| = 2. To show x on the number line, you need to show every number whose absolute value is 2. There are exactly two places where that happens: at 2 and at –2:

Now consider the inequality |x| > 2. To show x on the number line, you need to show every number whose absolute value is greater than 2. When you graph this on a number line, use open dots at –2 and 2 to indicate that those numbers are not part of the graph:

In general, you get two sets of values for any inequality |x| > k or |x| ≥ k, where k is any number.
There is only one exception to this statement. The graph of |x| ≥ k is the entire number line and thus can be thought of as just one set of values.
Now consider |x| ≤ 2. You are looking for numbers whose absolute values are less than or equal to 2. This is true for any number between 0 and 2, including both 0 and 2. It is also true for all of the opposite numbers between –2 and 0. When you graph this on a number line, the closed dots at –2 and 2 indicate that those numbers are included. This is due to the inequality using ≤ (less than or equal to) instead of < (less than).

In general, you only get one set of values for any inequality |x| < k or |x| ≤ k, where k is any number.
Again, there is only one exception. The graph of |x| < k is an empty graph with no values, as there are no values of x that makes the inequality true.
More generally, however, one way to think about it is that you’re still getting two sets of values (the “negative” set and the “positive” set), but because they meet at zero, they converge into one set. These inequalities can be rewritten without absolute value signs by writing the expression inside the absolute value as falling between two numbers:
- You can rewrite |x| < 2 like this: –2 < x < 2
- You can rewrite |x| ≤ 4 like this: –4 ≤ x ≤ 4
- You can rewrite |x + 6| < 25 like this: –25 < x + 6 < 25
Lesson 1: Introducing the concept
Materials: A number line and colored dots that the entire class can see
Preparation: If you don’t have a commercially prepared number line, draw one either on the board or on a long (preferably thin) sheet of paper. You could also share a digital absolute value number line. Include at least –20 to 20.
Standards:
- Interpret statements of inequality as the relative position of numbers on a number line. (Grade 6)
- Create and interpret statements of order for rational numbers in real-world contexts. (Grade 6)
Prerequisite skills and concepts: Students need to be familiar with the inequality symbols and how to make and use a number line. They also need to be able to compute with negative numbers.
- Stand so that the door is to your right.
- Ask: About how far am I from the door?
Students should respond with an estimated number of feet. - Ask: If I were blindfolded, how would you tell me where the door is?
Students should respond with both the estimated number of feet and a direction. If students provide different units, even nonstandard ones like “steps” or “arm lengths,” call attention to the differences and encourage them. - Now, stand so that the door is to your left.
- Ask: About how far am I from the door now?
Students should respond with an estimated number of feet. - Ask: If I were blindfolded, how would you tell me where the door is?
Students should respond with both the estimated length and a direction. - Say: When I asked how far I was from the door, you gave me a length, and it didn’t matter which way I was facing. But, when I asked where the door was in relation to my position, you gave me a direction as well as a number, and then it did matter which way I was facing. Today, we’ll discuss absolute value. Absolute value tells you how far a number is from zero. But it doesn’t tell you which way to go! It doesn’t tell you what direction a number is from zero.
- Point to 6 on the number line.
- Ask: What number is this? How far from zero is it?
Continue this questioning with –6, 0, 14, and –14, and so forth, until you are sure that students can differentiate between a directed distance and an absolute distance. Reinforce the response to each distance question by saying, ”The absolute value of [blank] is [blank]” and writing |___| = ___. - Divide the class into two teams. Team 1 is the Signed Numbers Team, and Team 2 is the Absolute Value Team.
- Ask: Can someone on Team 1 ask a question that would require a signed number—or a direction—as its answer?
You are looking for questions such as: What is the temperature in degrees Fahrenheit? How do I get to Lake Erie from here? If appropriate for you and your students, compare the different ways that signed numbers and direction appear in student answers. For example, a temperature value only has “direction” because degrees in Fahrenheit include negative and positive values, so a sign is needed for clarity. Directions to Lake Erie would require multiple signed numbers: where to travel east (positive) vs. west (negative) and where to travel north (positive) vs. south (negative). - Ask: Can someone on Team 2 ask the same question so that it does not require a signed number—or a direction—as its answer?
You are looking for questions such as: How far above zero degrees Fahrenheit is the temperature? How far north is Lake Erie from here? Have the teams alternate going first, thinking of questions that require a signed or absolute number to answer, and then having the other team revise the question. Encourage students to be creative with their questions. - Bring the full class together again. Place colored dots at –6 and +3 on the number line.
- Ask: How would you compare these two numbers? Elicit student responses and contrast differences in precision, order, and vocabulary. Ask students to both say and write the comparison: –6 < +3 or –6 < 3.
- Ask: How would you compare the absolute values of these two numbers? Ask students to both say and write the comparison. |–6| > |+3| or |–6| > |3|. Discuss why the direction of the comparison symbol changed, using the large number line to illustrate what the students and you say. Do several more examples until you are satisfied that students can compare both signed numbers and absolute values.
Lesson 2: Developing the concept
Materials: Index cards or digital “cards” that can be distributed among the class
Standards:
- Understand the absolute value of a rational number as its distance from 0 on the number line. (Grade 6)
Preparation: Make cards for I Have . . . Who Has?, explained in the assessment game below.
- Say: Remember that absolute value is the distance that a number is from 0, no matter which direction.
- Ask: Can someone write an equation that means “24 is the absolute value of the number that is 6 less than x?”
The equation, 24 = |x – 6|, represents the situation. You may need to repeat the equation several times, slowly, as students try to parse it out. - Ask: What can be the value of the expression inside the absolute value symbols?
It is natural to show that x – 6 can have a value of 24. Help students see that the expression can also have a value of –24. If necessary, remind them of your previous discussion about directed distance from zero as opposed to absolute distance from zero. - Ask: If the expression can have a value of 24 or –24, what values can x have?
Have students try to find possible values for x themselves at first. Then have them compare what they found, and facilitate a discussion around different strategies they used.
If x = 30, then x – 6 = 24. If x = –18, then x – 6 = –24. There are two possible values for x: 30 and –18. - Repeat the last three questions using a variety of absolute value expressions:
- |13 – x| = 14 (Solution: x = –1 or x = 27)
- |25 + x| = 25 (Solution: x = 0 or x = –50)
- 42 = |2x| (Solution: x = 21 or x = –21)
- 1 = |x ÷ 36| (Solution: x = 36 or x = –36)
- 0 = |36 ÷ x| (Solution: There is no value for x that satisfies this equation.)
Wrap-up and assessment game
- Ask students to write and share their own definitions and real-life examples of absolute value situations.
- Play I Have . . . Who Has? Make up a set of 15 index cards with absolute value equations and 15 index cards containing values for the variable. Alternatively, create a way for the 30 equations below to be distributed as equally as possible among your students, whether physically or digitally.
| Absolute Value Cards | Variable Value Cards |
| |x + 5| = 20 | x = 15 |
| |5 – x| = 30 | x = –25 |
| |x + 6| = 41 | x = 35 |
| |–27 – x| = 20 | x = –47 |
| –7 + |x| = 0 | x = –7 |
| |25 – x| = 18 | x = 7 |
| |x + –5| = 38 | x = 43 |
| |37 – x| = 70 | x = –33 |
| 114 – |x| = 7 | x = 107 |
| |–x + 100| = 21 | x = 121 |
| –|1 + x| = -80 | x = 79 |
| |x| = 81 | x = –81 |
| |x + 3| = 84 | x = 81 |
| |25 + x| = 62 | x = –87 |
| |x – 26| = 11 | x = 37 |
Each Absolute Value Card listed has two values for x. These values overlap so that each Variable Value Card satisfies two of the given absolute value equations (the first and second values satisfy the first equation, the second and third values satisfy the second equation, and so on, until the last and first values satisfy the last equation).
Distribute the cards or equations equally. Be sure they’ve all been distributed. If necessary, give some students multiple equations. Choose a student to say “I have” and read the equation on their card. Then have the student say “Who has a match?” Any student with a match should say “I Have . . . Who Has," and the game proceeds until all cards have been read. You might have students stand when the game starts and sit as they offer a response. To keep all engaged, offer a reward for successful completion of the game and encourage challenges to suspect responses.
***
This blog post, originally published in 2021, has been updated for 2024.
Looking for a math curriculum that will grow student confidence in mathematics and provide you with rich lessons and activities for middle school students? Explore HMH Into Math, our core math solution for Grades K–8.
After teaching about positive numbers, hear our podcast episode on having a positive math identity.














