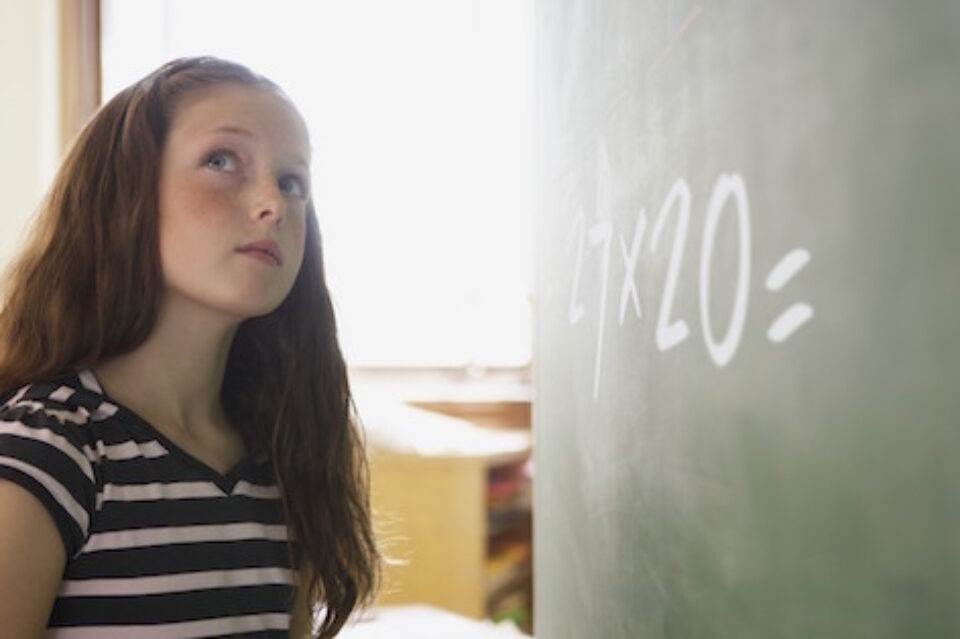
Number sense, like commonsense, is easy to recognize, but not always easy to describe or support. In a world of computers and handheld devices, good number sense is more important today than at any time. As Keith Devlin, Stanford mathematician, wrote, "The most basic of today’s new mathematical skills is number sense. (The other important one is mathematical thinking.) But whereas the latter is important for those going into STEM careers, number sense is a crucial 21st-century life skill for everyone" (Huffington Post, 2017).
Moreover, we now know that number sense begins in the first years of life; if not developed in school, a lack of number sense can cause students to struggle in the upper grades and in college.
What is number sense?
Number sense refers to the ability to understand numbers and how they relate and connect with one another other. Those with a good number sense can do the following.
- compose and decompose numbers to solve calculations
- compute mentally using a variety of strategies
- make reasonable estimates
- understand quantity and magnitude
- reason with numbers
What does it mean to decompose and compose numbers to calculate? Let’s look at an example. In a fifth grade class, students were solving a word problem that required the product of 25 x 26. Instead of assuming everyone would find the product in the same way, students are encouraged to find a variety of methods.
Before you read on, think of some ways you might multiply 25 x 26. Students may have a variety of responses.
One might say 25 x 26 is the same as 26 x 25.
Another might think of 26 as 20 + 6 and calculate the following:
20 x 25 = 500
6 x 25 = 150
26 x 25 = 650
Another may say that they did it similarly but instead of 20, multiplied by 10 twice. Still another suggested that beginning with 30 x 25 was easier than 26 x 25:
30 x 25 = 750, but that is four 25’s too many
4 x 25 = 100
750 -100 = 650
Some other solutions may include thinking of it in quarters:
4 x 25 = 100
26 ÷ 4 = 6.5
6.5 x 100 = 650
One student may suggest that 25 x 26 was too difficult, but it was the same as 13 x 50 because they doubled the 25 and halved the 26.
13 x 50 = (10 x 50) + (3 x 50 ) = 500 + 150 = 650
Finally one student may have studied a table of square numbers in the room and saw that 25 x 25 = 625 and 1 more 25 is 650.
Nurturing number sense in the classroom
Of course this variety and number of strategies doesn't occur naturally, but only in classrooms where students are encouraged to take risks, to look for structures like doubling and halving factors, to use known facts to figure out unknown ones, and most of all to share and examine each others’ thinking. Students work in pairs to come up with solutions, first with easier problems (think 11 x 15) then with those of increasing complexity.
Student solutions are posted, discussed, and whenever possible, generalized. (26 = 20 + 6 or knowing that 4 x 25 equals 100 enables you to multiply by 100 and divide by 4 when multiplying by 25.)
Finally, providing visual models for procedures deepens understanding. For instance an area model helps students understand the distributive property.
20 x 25 = 500
6 x 25 = 150
26 x 25 = 500 + 150 = 650

Is the answer the same as one derived with the paper pencil algorithm? Of course, but which develops number sense? Which encourages students to use properties like the commutative and distributive property? Which enables students to solve more complicated problems mentally?
Think how different it would be if students merely wrote 25 x 26 vertically and then did something like this:
26
x 25
5 x 6 = 30, carry the 3, put down 0, 5 x 2 = 10, add 3 = 13. And so on. No number sense. No place value. No idea if the answer is reasonable.
Taking the time to thoroughly examine one or two calculations in depth, similar to the above, encourages students to focus on thinking—not merely on getting the correct answer. It leads both to number sense and to underlying math concepts.
The views expressed in this article are those of the author and do not necessarily represent those of HMH.
***
Grow student confidence in mathematics with HMH Into Math, our core math solution for Grades K–8.
This blog post, originally published in 2017, has been updated for 2025.
Get our FREE guide “Optimizing the Math Classroom: 6 Best Practices.”














